This paper is available on arxiv under CC 4.0 license.
Authors:
(1) Macoto Kikuchi (菊池誠), Cybermedia center, Osaka University, and Department of Physics, Osaka University and [email protected].
Table of Links
IV. DISCUSSION
The results presented in this paper suggest that the one-way switches are hardly selected in evolution because mutationally robust GNRs are favored. This scenario of the phenotype selection was confirmed by comparing the outcomes of the multicanonical ensemble method and the steady state of stochastic evolutionary simulations because
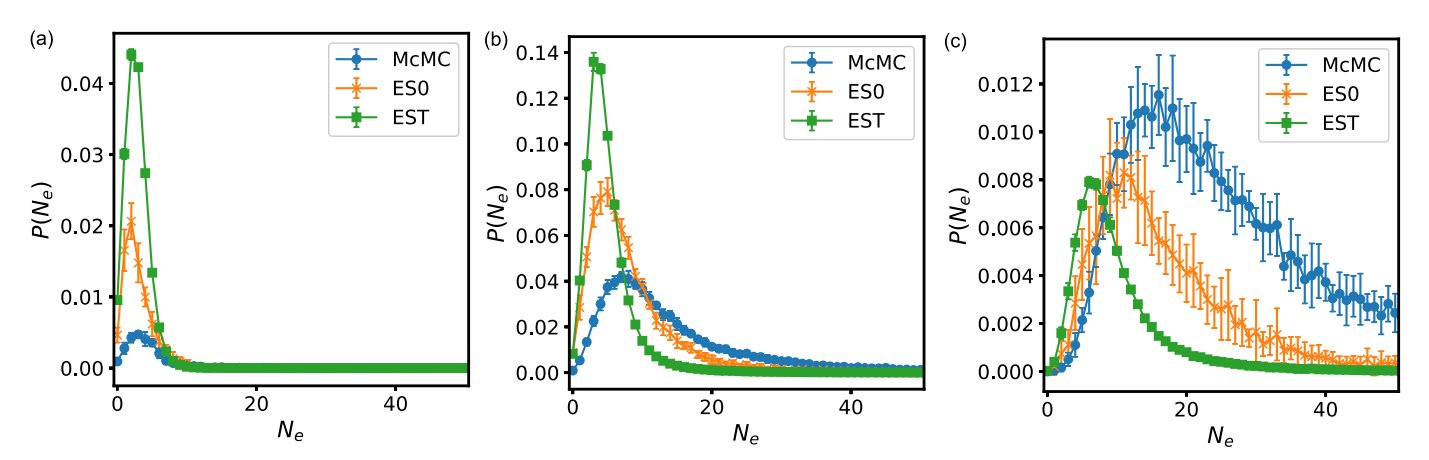
the steady state does not depend on the evolutionary history. We consider this selection mechanism to be widely valid and not restricted to the case studied in this paper. Since this phenotype selection can occur whenever more than one phenotype exists for the same fitness value, we expect this phenomenon to occur in real living systems. We may call it the third-order selection because it is a consequence of the second-order selection.
Since the second-order selection is a weak effect, the above phenotypic selection may be a further weak effect. However, as the present results suggest, the phenomenon may become significant if the steady state of evolution is maintained. Such situations can be realized experimentally. For example, it will be possible for the effect to be observed for bacteria under constant stress. It should be noted that what is the matter is not the absolute strength of mutational robustness but its relative strength. Thus, phenotype selection can occur even between mutationally not-so-robust phenotypes.
Next, let us consider an implication of the evolution of the model dealt with observed in this paper. The result of McMC shows that most GRNs are monostable at low fitness; then as fitness increases the toggle switches dominate, and eventually most GRNs become one-way switches. A one-way switch of GRN realizes an irreversible change in the cell states. We note that irreversibility was not required in the definition of fitness. Thus, the irreversible switches can emerge even without it being required explicitly as fitness. It suggests the possibility that the irreversibility observed for real cells has evolved from the monostable reversible GRNs.
Finally, the present study shows that McMC is an effective method for investigating characteristic properties of evolution. McMC clarifies what kind of phenotypes can exist if the evolution process is not considered. The fact that the outcomes of McMC and evolutionary simulation differ considerably implies a possibility that some phenotypes are not reached by evolution. Considering the experimental situation, such phenotypes may be accessible by direct genome engineering other than evolutionary experiments. Apart from the evolution of living systems, the present results give an insight into the genetic algorithm (GA) of optimization problems; GA is not a fair method for optimization; rather, it will provide the phenotypes bearing a specific property, namely, the mutational robustness, as the optimized solutions.
ACKNOWLEDGMENTS
This work was supported by JSPS KAKENHI Grant Number 23K03261.
[1] H. Kitano, Biological robustness, Nat Rev Genet 5, 828 (2004).
[2] A. Wagner, Robustness and Evolvability in Living Systems (Princeton Univ Press, 2005).
[3] J. Masel and M. L. Siegal, Robustness: mechanisms and consequences, Trends Genet 25, 395 (2009).
[4] G. Giaever, A. M. Chu, L. Ni, C. Connelly, L. Riles, S. Veronneau, S. Dow, A. Lucau-Danila, K. Anderson, B. Andre, A. P. Arkin, A. Astromoff, M. E. Bakkoury, R. Bangham, R. Benito, S. Brachat, S. Campanaro, M. Curtiss, K. Davis, A. Deutschbauer, K. D. Entian, P. Flaherty, F. Foury, D. J. Garfinkel, M. Gerstein, D. Gotte, U. Gludener, J. H. Hegemann, S. Hempel, Z. Herman, D. F. Jaramillo, D. E. Kelly, S. L. Kelly, P. Kotter, D. LaBonte, D. C. Lamb, N. Lan, H. Liang, H. Liao, L. Liu, C. Luo, M. Lussier, R. Mao, P. Menard, S. L. Ooi, J. L. Revuelta, C. J. Roberts, M. Rose, P. RossMacdonald, B. Scherens, G. Schimmack, B. Shafer, D. D. Shoemaker, S. Sookhai-Mahadeo, R. K. Storms, J. N. Strathern, G. Valle1, M. Voet, G. Volckaert, C. Y. Wang, T. R. Ward, J. Wilhelmy, E. A. Winzeler, Y. Yang, G. Yen, E. Youngman, K. Yu, H. Bussey, J. D. Boeke, M. Snyder, P. Philippsen, R. W. Davis, and M. Johnston, Functional profiling of the saccharomyces cerevisiae genome, Nature 418, 387 (2002).
[5] T. Baba, T. Ara, M. Hasegawa, Y. Takai, Y. Okumura, M. Baba, K. A. Datsenko, M. Tomita, B. L. Wanner, and H. Mori, Construction of escherichia coli k-12 in-frame, single-gene knockout mutants: the keio collection, Mol Syst Biol 2, 2006.0008 (2006).
[6] D. U. Kim, J. Hayles, D. Kim, V. Wood, H. O. Park, M. Won, H. S. Yoo, T. Duhig, M. Nam, G. Palmer, S. Han, L. Jeffery, S. T. Baek, H. Lee, Y. S. Shim, M. Lee, L. K. and. K. S. Heo, E. J. Noh, A. R. Lee, Y. J. Jang, K. S. Chung, S. J. Choi, J. Y. Park, Y. Park, H. M. Kim, S. K. Park, H. J. Park, E. J. Kang, H. B. Kim, H. S. Kang, H. M. Park, K. Kim, K. Song, K. B. Song, P. Nurse, and K. L. Hoe, Analysis of a genome-wide set of gene deletions in the fission yeast schizosaccharomyces pombe, Nat Biotechnol 28, 617 (2010).
[7] M. Isaran, C. Lemerle, K. Michalodimitrakis, C. Horn, P. Beltrao, E. Raineri, M. Garriga-Canut, and L. Serrano, Evolvability and hierarchy in rewired bacterial gene networks, Nature 452, 840 (2008).
[8] E. van Nimwegen, J. P. Crutchfield, and M. Huynen, Neutral evolution of mutational robustness, Proc Natl Acad Sci USA 17, 9716 (1999).
[9] S. Ciliberti, O. C. Martin, and A. Wagner, Robustness can evolve gradually in complex regulatory gene networks with varying topology, PLoS Comput Biol 3, e15 (2007).
[10] S. Ciliberti, O. C. Martin, and A. Wagner, Innovation and robustness in complex regulatory gene networks, Proc Natl Acad Sci USA 104, 13591 (2007).
[11] B. A. Berg and T. Neuhaus, Multicanonical algorithms for first order phase transitions, Phys Lett B267, 249 (1991).
[12] B. A. Berg and T. Neuhaus, Multicanonical ensemble: A new approach to simulate first-order phase transitions, Phys Rev Lett 68, 9 (1992).
[13] Y. Iba, N. Saito, and A. Kitajima, Multicanonical mcmc for sampling rare events: an illustrative review, Ann Inst Stat Math 66, 611 (2014).
[14] A. Kitajima and M. Kikuchi, Numerous but rare: An exploration of magic squares, PLoS ONE 10, e0125062 (2015).
[15] N. Saito and M. Kikuchi, Robustness leads close to the edge of chaos in coupled map networks: toward the understanding of biological networks, New J Phys 15, 053037 (2013).
[16] S. Nagata and M. Kikuchi, Emergence of cooperative bistability and robustness of gene regulatory networks, PLoS Comput Biol 16, e1007969 (2020).
[17] T. Kaneko and M. Kikuchi, Evolution enhances mutational robustness and suppresses the emergence of a new phenotype: A new computational approach for studying evolution, PLoS Comput Biol 18, e1009796 (2022).
[18] Y. Omachi, N. Saito, and C. Furusawa, Rare-event sampling analysis uncovers the fitness landscape of the genetic code, PLoS Comput Biol 19, e1011034 (2023).
[19] J. J. Tyson, K. C. Chen, and B. Novak, Sniffers, buzzers, toggles and blinkers: dynamics of regulatory and signaling pathways in the cell, Curr Opin Cell Biol 15, 221 (2003).
[20] J. E. Ferrell and E. M. Machleder, The biochemical bases of an all-or-none cell fate switch in xenopus oocytes, Science 280, 895 (1998).
[21] E. Mjolsness, D. H. Sharp, and J. Reinitz, A connectionist model of development, J Theor Biol 152, 429 (1991).
[22] A. Wagner, Evolution of gene networks by gene duplications: A mathematical model and its implications on genome organization, Proc Natl Acad Sci USA 91, 4387 (1994).
[23] A. Wagner, Does evolutionary plasticity evolve, Evolution 50, 1008 (1996).
[24] M. L. Siegal and A. Bergman, Waddington’s canalization revisited: developmental stability and evolution, Proc Natl Acad Sci USA 99, 10528 (2002).
[25] J. Masel, Genetic assimilation can occur in the absence of selection for the assimilating phenotype, suggesting a role for the canalization heuristic, J Evol Biol 17, 1106 (2004).
[26] K. Kaneko, Evolution of robustness to noise and mutation in gene expression dynamics, PLoS one 2, e434 (2007).
[27] C. Espinosa-Soto, O. C. Martin, and A. Wagner, Phenotypic robustness can increase phenotypic variability after nongenetic perturbations in gene regulatory circuits, J Evol Biol 24, 1284 (2011).
[28] M. Inoue and K. Kaneko, Cooperative adaptive responses in gene regulatory networks with many degrees of freedom, PLoS Comput Biol 9, e1003001 (2013).
[29] M. Inoue and K. Kaneko, Cooperative reliable response from sloppy gene-expression dynamics, Eutophys Lett 124, 38002 (2018).
[30] F. Wang and D. P. Landau, Efficient, multiple-range random walk algorithm to calculate the density of state, Phys Rev Lett 86, 2050 (2001).
[31] F. Wang and D. P. Landau, Determining the density of states for classical statistical models: A random walk algorithm to produce a flat histogram, Phys Rev E 64, 056101 (2001).
[32] J. Lee, New monte carlo algorithm: Entropic sampling, Phys Rev Lett 71, 211 (1993).

